EN224: Linear Elasticity

 

Homework 4: Complex variable methods for anti-plane shear problems

Due Friday April 8, 2005

 

 

Division of Engineering

Brown University

 

1. Consider the analytic function , with a branch cut along the negative real axis.  Find expressions for the anti-plane shear stress and displacement fields generated by this potential, and hence identify the anti-plane shear boundary value problem that this potential solves.

 

2. Anti-plane shear solution to rigid circular inclusion in an infinite solid.

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

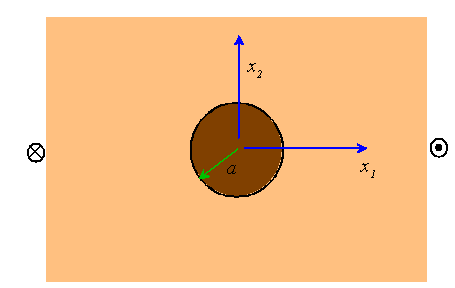
An infinite solid contains a rigid cylindrical inclusion of radius a at the origin.  The solid is subjected to remote anti-plane shear loading:

By expanding the potential in a Laurent series, find the complex potential f(z)  such that

 .  Hence, find the displacement and stress fields.

 

 

3. Hypotrochoidal hole in an infinite solid. The mapping

maps the unit circle onto a shape that resembles (vaguely) a square. Clearly, a just makes the square larger or smaller.  Plot the image of |z|=1 under this mapping for a few values of  and  to figure out what they do (keep  small or you will get something weird).

Suppose that an infinite solid contains a hole whose boundary is the image of the unit circle under this mapping.  Assume that the solid is subjected to remote anti-plane shear loading as in Problem 3. Find the state of stress in the solid. (you can leave your answer in terms of    ).

Find the greatest stress concentration factor (you can use your intuition to figure out what orientation of the hole will lead to the greatest stress concentration, you don’t have to prove it).

Plot some stress and displacement contours using your favorite symbolic manipulation program (Maple has a useful `transform’ function in the plottools package that makes this very simple)