PROVIDENCE, R.I. [Brown University] — A new measurement of heat conduction in an exotic state of matter points to the presence of strange particles that could be useful in quantum computers.
In a paper published this week in the journal Nature, a research team including a Brown University physicist has characterized how heat is conducted in a matter state known as a quantum Hall liquid, in which electrons are confined to two dimensions. The findings suggest the presence of non-Abelian anyons, quantum quasi-particles that retain a “memory” of their relative positions in the past. Theorists have suggested that the ability of these particles to retain information could be useful in developing ultra-fast quantum computing systems that don’t require error correction, which is a major stumbling block in the development of quantum computers.
The research was led by an experimental group at the Weizmann Institute of Science in Rehovot, Israel. Dmitri Feldman, a professor of physics at Brown, was part of the research group. He discussed the findings in an interview.
Q: Could you explain more about what you and your colleagues found?
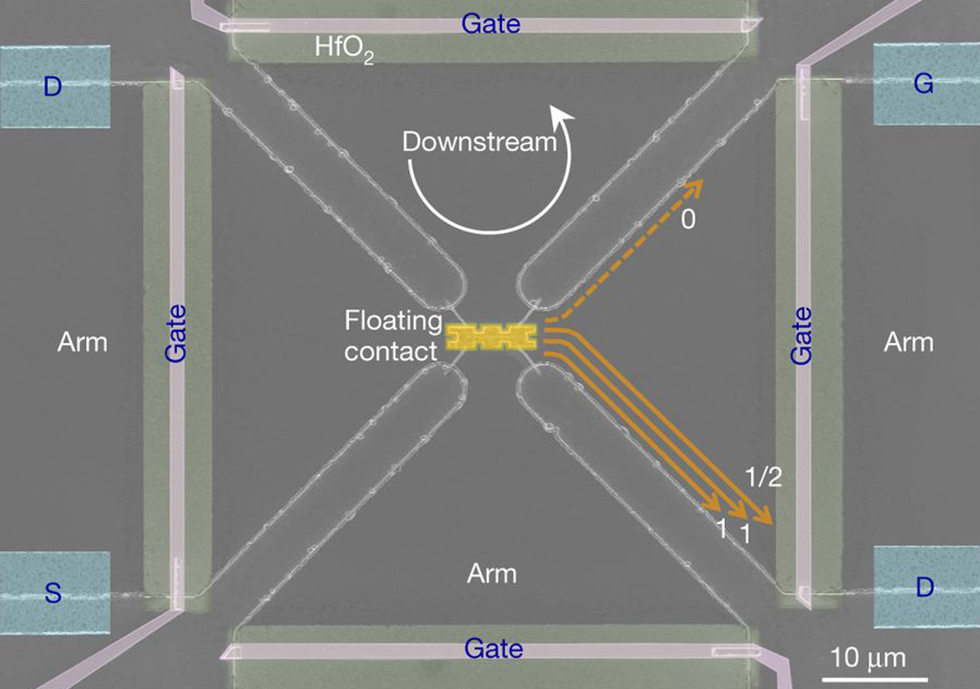
A: We were looking at thermal conductance — which simply means the flow of heat from a higher temperature to a lower temperature — in what’s known as a 5/2 quantum Hall liquid. Quantum Hall liquids are not ‘liquids’ in the conventional sense of the word. The term refers to the behavior of electrons inside certain materials when the electrons become confined in two dimensions in a strong magnetic field.
What we found was that the quantized heat conductance — meaning a fundamental unit of conductance — in this system is fractional. In other words, the value was not an integer, and that has interesting implications for what’s happening in the system. When the quantum thermal conductance is not an integer, it means that quasi-particles known as non-Abelian anyons are present in this system.
Q: Can you explain more about non-Abelian anyons?
A: In the Standard Model of particle physics, there are only two categories of particles: fermions and bosons. That’s all there is in the world we experience on a daily basis. But in two-dimensional systems like quantum Hall liquids, there can be other types of particles known as anyons. Generally speaking, there are two types of anyons: Abelian anyons and non-Abelian anyons. Abelian anyons behave more or less like conventional fermions, but non-Abelian anyons are, for lack of a better way of saying it, completely insane. They have very strange properties that could be used in quantum computing, or more specifically, for what’s known as topological quantum memory.
Q: What’s the connection between non-Abelian anyons and quantum computing?
A: A regular quantum computer — one without non-Abelian anyons — would require error correction. For one useful quantum bit of information, you need multiple additional quantum bits to correct errors that arise from random fluctuations in the system. That’s extremely demanding and a big problem in quantum computing. But topological quantum computing — which requires the presence of non-Abelian anyons — is unique in that it doesn’t need error correction to make the quantum bits useful. That’s because in a non-Abelian system, you can produce states that are completely indistinguishable locally, but globally the states are completely different. So you can have random perturbations of these local quantum numbers, but it won’t change the global quantum numbers, which means the information is safe.
Q: Where does this line of research go from here?
A: This work suggests that a particular entity known as a Majorana particle is at work in the particular system that we studied. And that suggests that a Majorana-based quantum computer is possible. But an even more powerful computational platform would come from what’s known as parafermions, which have been theorized but not yet shown to exist. Perhaps their existence could also be proven with similar experimental tools in the future.